Cometary Science Archive

ECLIPSE CENTRAL

Helpful Resources and Information for 2017 August 21 Total Solar Eclipse

A total solar eclipse is one of the most amazing spectacles of nature. One must be inside a relatively narrow path as the moon's umbral shadow speeds along the surface of the earth at 1600-1700 mph. If inside this path, for a short period (seconds, several minutes), and if clear, you can see the moon blot out all of the sun's harmful rays, producing a twilight scene with the brightest stars visible along with the beautiful outer atmosphere of the sun (known as the corona) and often prominences (storms that can be very red in color) just peering over the lunar limb.

The path of totality is rarely more than 300 km wide, but the penumbra shadow (in which partial eclipse can be seen) is about 7000 km wide. Thus, one can see many partial solar eclipses in a lifetime without moving (in which one must use filters or projection to safely view the partially covered sun), but most people have to travel some distance to see totality in their lifetime.

Some maps depicting the path of totality on Monday, 2017 August 21:

Interactive Google map, via NASA, for 2017 August 21 total eclipse

You can zoom in or out, as needed. Clicking on any point on the map will give the duration of totality (2m40s maximum), the sun's altitude and azimuth in the sky, and the times for various phases of the eclipse in Universal Time (UT). Note that EDT = UT - 4 hours; CDT = UT - 5 hours; MDT = UT - 6 hours; and PDT = UT - 7 hours. One must be to be inside the two parallel blue lines (which are about 60 miles apart) and as close to the red central line as possible, to see the longest duration of the moon totally covering the sun's disk.

NASA eclipse map of the entire earth, for 2017 August 21 total eclipse

Here you see the path of totality in narrow, parallel, blue lines; the green lines parallel to these show how much the sun will be eclipsed in partial eclipse as you move away from the totality path -- 0.80 meaning 80% of the sun cover, 0.60 meaning 60% covered, etc. (thus, New York City and Las Vegas saw about three-quarters of the sun covered at peak).

This NASA animation shows the shadow of the moon passing over the earth -- all areas inside that shadow saw a partial eclipse, but only viewers near the small dot moving across the central US from west to east saw the sun totally eclipsed by the moon.

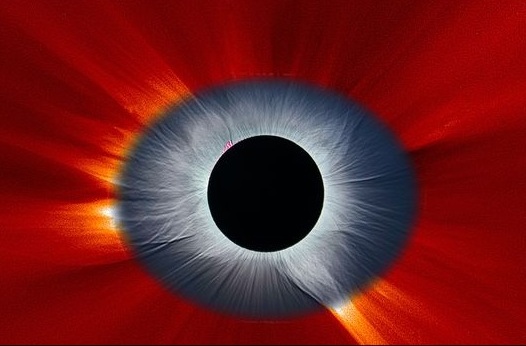
Photo of the 2017 August 21 total solar eclipse, showing spectacular corona, taken by Pei-Yuan Sun at Madras, OR, USA (sent courtesy of Tao Chen of Suzhou, China).

Some statistics about solar eclipses:

The sun's apparent diameter ranges from 31'.47 (31.47 arc minutes) at aphelion to 32'.53 at perihelion. The moon's apparent diameter ranges from 29'.4 to 33'.53. [There are 60 arc minutes on one degree, so the sun and moon are roughly half a degree in size.] Thus, the moon's apparent diameter can exceed the sun's by as much as 2' or can be as much as 3' smaller. It's a happy coincidence for us that -- though the sun is 1,400,000 km across and the moon is only 3500 km in diameter -- the sun is 400 times farther than the moon (to compensate for it' being 400 times larger).

The moon's orbit is inclined about 5 degrees with respect to the earth's orbit about the sun, so though the moon lines up with the sun every 29.5 days or so, it isn't always in a direct line. Thus, total solar eclipses only occur somewhere on the earth approximately every 1.5 years.

From 2001 to 2100, there are 224 solar eclipses, of which 77 are partial only, 70 are annular, 67 are total, and 7 are hybrid (annual/total mixed). Total eclipses can last as long as seven and a half minutes; annual eclipses can last as long as twelve and a half minutes.

Eclipses of similar duration repeat in a "saros" cycle of approximately 18 years 11 days, each time shifting about a third of the way around the world westward and about 4 or 5 degrees north or south in geographical latitude. A series of eclipses from one saros begins at one pole and ends at the opposite pole some 12-15 centuries later. New saros eclipses are beginning as old saros cycles end. Ancient astronomers learned of both the saros cycle and the metonic cycle, the latter being a cycle in which non-saros-group eclipses occur almost exactly every 19 years (thus, eclipses of different durations and not-predicatable locations on the earth with respect to each other). The eclipses of 1979 Feb. 26 and 1998 Feb. 26 are an example of the metonic cycle.

Definitions:

Eclipse magnitude = the fraction of the sun's diameter occulted by the moon.

UT = Universal Time (in USA, EDT = UT - 4 hours; CDT = UT - 5 hr; MDT = UT - 6 hr; PDT = UT - 7 hours).

Information on the total solar eclipse of 2024 April 8

On 2024 April 8, totality will again cross the USA, this time from southwest to northeast, through Mexico, Texas, and up over Niagara Falls and into northern New England and northeast Canada. An interactive Google map of the path of totality is available here.

Historical maps of solar eclipses

All solar eclipse paths projected onto the earth, by double-decades:

[from F. Espenak (1987), *Fifty Year Canon of Solar Eclipses: 1986-2035*, NASA Ref. Publ. 1178 Revised. Has the north-polar diagram showing eclipses of Saros 120. Has global (in 1/3 segments) maps of eclipses at 20-yr intervals]

Eclipse paths for individual eclipses, 1969-2032:

[from F. Espenak (2006), *Five Millennium Canon of Solar Eclipses: -1999 to +3000 (2000 BCE to 3000 CE)*, NASA/TP-2006-214141]

Tables of solar-eclipse tables, 1987 to 2041

[from F. Espenak and J. Meeus (2009), *Five Millennium Canon of Solar Eclipses: -1999 to +3000 (2000 BCE to 3000 CE) -- Revised*, NASA/TP-2009-214174)]:

The earth's rotation has been slowing down with time (currently at a rate of about 84 seconds in a century), due to the effects of the moving oceans and the gravitational forces of the moon and sun; this energy is transferred to the moon, causing its orbit to slowly move away from the earth. A leap second is thus now introduced about once every 12-18 months or so. Delta(T) = TDT - UT = atomic-clock time minus observed time with respect to the stars/sun. Two thousand years ago, Delta(T) was about 2.92 hours, or 2h55m [diagram from *Historical Eclipses and Earth's Rotation*, by F. R. Stephenson (1997, Cambridge University Press].

This diagram [also from Stephenson (1997)] shows what happens with a predicted eclipse in the distant past when no allowance is made for the slowing of the earth's rotation.

Other excellent eclipse websites:

  • Fred Espenak's website [explanations with diagrams of eclipses; links to historical and future eclipse maps and photographs]
  • Fred Espenak's secondary website [focus on historical photos and reports, plus links to instructional webpages and to historical and future eclipses]

The CSL/CSC and CBAT use computers generously supplied by the Tamkin Foundation.

About This Service

Here are credits and a user-feedback form.

Further information is available via e-mail from icq(at)cfa.harvard.edu